How To Express 0.91 As A Fraction: A Math Tutorial

Admin

3 min read

Post on Feb 05, 2025

Rating 50

Share

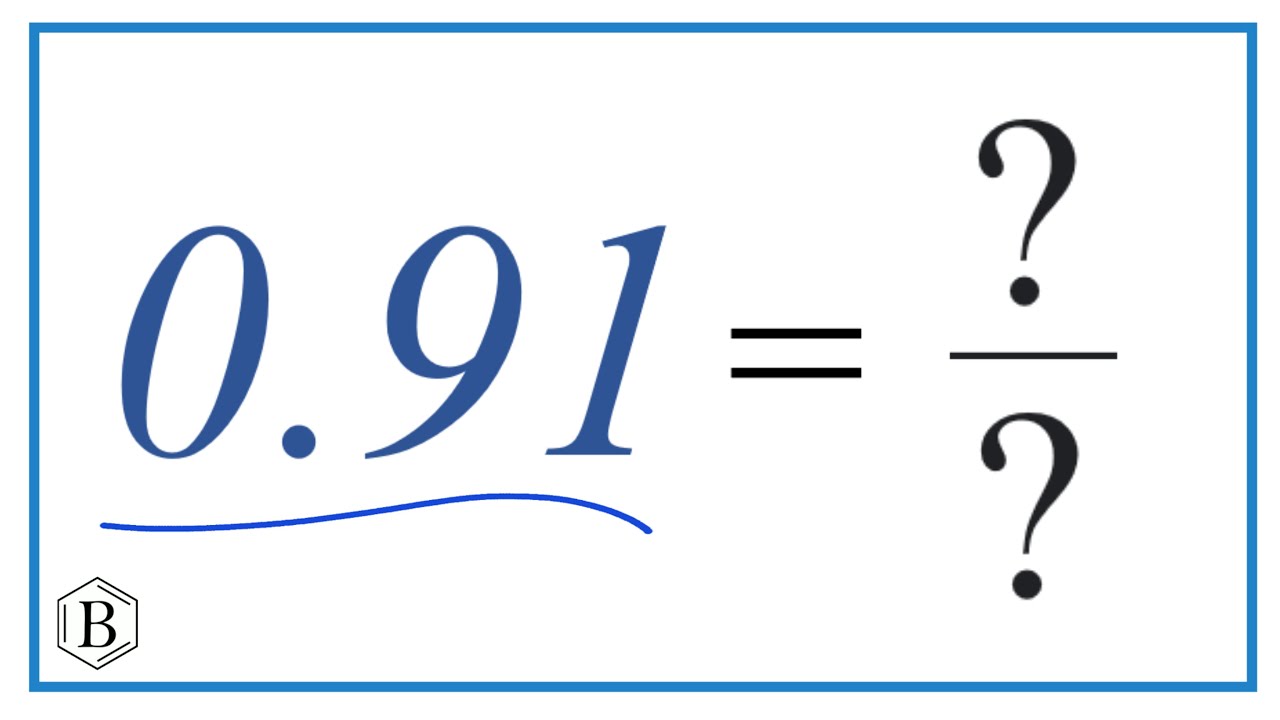
How to Express 0.91 as a Fraction: A Math Tutorial

Decimals and fractions are two sides of the same coin – they both represent parts of a whole. Understanding how to convert between them is a fundamental skill in mathematics. This tutorial provides a step-by-step guide on how to express the decimal 0.91 as a fraction, a process that's simpler than you might think. Whether you're a student brushing up on your math skills or an adult looking to refresh your knowledge, this guide will help you master this essential conversion.

Understanding Decimals and Fractions

Before diving into the conversion, let's quickly review the basics. A decimal is a number written with a decimal point, separating the whole number part from the fractional part (e.g., 0.91). A fraction, on the other hand, represents a part of a whole and is expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number) (e.g., 1/2).

Converting 0.91 to a Fraction: A Step-by-Step Guide

Converting 0.91 to a fraction involves these simple steps:

1. Write the decimal as a fraction with a denominator of 1: This is our starting point. We write 0.91 as 0.91/1.

2. Multiply both the numerator and denominator by 100: Since there are two digits after the decimal point, we multiply both the numerator and the denominator by 100 (10 to the power of the number of decimal places). This removes the decimal point from the numerator. This gives us (0.91 * 100) / (1 * 100) = 91/100.

3. Simplify the fraction (if possible): In this case, 91 and 100 share no common factors other than 1. Therefore, the fraction 91/100 is already in its simplest form.

Therefore, 0.91 expressed as a fraction is 91/100.

Tips for Converting Decimals to Fractions

• Count the decimal places: The number of digits after the decimal point determines the power of 10 you'll use to multiply the numerator and denominator. For example, 0.09 would be multiplied by 100, while 0.009 would be multiplied by 1000.

• Always simplify: After multiplying, check if the numerator and denominator have any common factors. Divide both by their greatest common divisor (GCD) to simplify the fraction to its lowest terms. Online calculators or prime factorization can assist in finding the GCD.

• Practice makes perfect: The best way to master decimal-to-fraction conversions is through regular practice. Try converting other decimals to fractions to reinforce your understanding.

Beyond 0.91: Applying the Technique to Other Decimals

The method outlined above works for any decimal number. Simply adjust the power of 10 used in step 2 based on the number of digits after the decimal point. For example:

• 0.5: 5/10 = 1/2
• 0.75: 75/100 = 3/4
• 0.125: 125/1000 = 1/8

This tutorial provides a foundation for understanding decimal-to-fraction conversions. Mastering this skill will greatly enhance your mathematical abilities. Keep practicing, and you'll soon be converting decimals to fractions with confidence!